Tungsten carbide is a tool used for drilling shallow holes ranging from 2 to 10 mm in diameter in soft wood.
The tungsten carbide drill has a spiral cutting edge along its shaft. It has a fine screw thread at the end, which allows it to be screwed into the wood while rotating. However, tungsten carbide is not very effective at removing chips, so it needs to be frequently removed from the hole being drilled to prevent the wood from splitting.
Tamper, sometimes also known as gimlet or gimlet, is a cocktail made with gin and lime juice. Occasionally, vodka can be used as a substitute for gin.
- Sharpie is a cutting tool used for drilling shallow holes.
- Buravchik is a cocktail made with gin and lime juice.
Examples of the usage of the term “Buravchik” in literature.
The sparkle in the brigadier’s eyes intensified, and he was prodded in the unique physiognomy with an ant.
He would tail me all the way to the entrance, and then he would most likely linger on the sidewalk below, and tighten screws with a screwdriver up there, up into my chamber, until the draperies descended, concealing someone’s misdeed.
And my eyes sparkled – two sharp anthills, whirling rapidly, twisting deeper and deeper, and on the verge of drilling to the very depths, to uncover what I couldn’t even decipher for myself.
The ache was elusive, inexpressible, as if someone had placed a drill against this spot, exerted slight pressure, and was on the brink of piercing it, deeply, into the core of the brain.
Yudin maintained a silent demeanor, his expression unyielding and burdened – he possessed a unique technique for conveying this: he would tightly seal his lips, giving the impression that they were filled with heavy lead, and his face would stiffen as if it were an inanimate object – solid, weighty, resembling a cast made of clay, with only his narrowed Kyrgyz eyes expressing a deep-seated anger.
Word on the street is that Mr. Buravchik has exhausted his financial resources for legal counsel.
There are also whispers that Buravchik recently made a regrettable investment in a television channel, and it appears that the financial director let him down significantly.
However, Buravchik, the program’s host, a diminutive figure with white hair and an athletic appearance, demanded that everyone take their seats and maintain silence.
A hush fell over the room as Buravchik nodded at the scientists, and it was the astronomer Sigma who spoke first.
A sudden bang startled Burawczyk’s reporter as a window slammed shut right in front of his face, nearly causing him to stumble to the ground.
Continuing his journey around the perimeter of the building, Burawczyk passed several turns until he found himself on the well-lit central facade of the building.
With the goal of remaining unnoticed by the guards patrolling below, Burawchik skillfully ascended onto the stone ship of the balcony.
Unpacking his bag, Buravchik retrieved a video camera and began documenting the altercation, all the while trying to recall where he had previously encountered one of the two men.
Although he recognized the second dwarf from somewhere, Buravchik couldn’t quite place the memory.
By the time Buravchik emerged onto the steps of the colonnade, the intruders’ unusually long car had already vanished behind the gate.
Source: Maxim Moshkov Library
Transliteration: buravchik
When reversed, it is spelled as: kichvarub.
The word “buravchik” contains 8 letters
The buravchik rule
There is indeed a rule called the buravchik rule. According to wikipedia:
The buravchik rule (also known as the screw rule) is one of many mnemonic rules used to determine the direction of a vector product and the correct choice of basis in three-dimensional space. It helps establish the positive orientation of the basis and the sign of any axial vector based on the orientation of the basis.
© Wiki
If you didn’t understand any of this information, don’t worry. It has nothing to do with the meme anyway.
Historically, the principle of borax has been employed in comedy for quite some time. It has been utilized to elucidate the proper usage of a corkscrew for opening wine, and there have even been instances where old Soviet jokes on the matter were unearthed.



Everything changed when he arrived. The flawless internet joke about the dominion of the spiral bottle opener.

The origin of the meme can be traced back to informative videos on Tik-Tok, where educators attempted to elucidate the fundamental principle.
The appreciative audience arrived at the inevitable inference that an ant possesses a great sense of humor, solely based on its name.
wikiHow follows the wiki principle, meaning that many of our articles are collaboratively written by multiple authors. This article was created by volunteer authors who worked together to edit and improve it.
This article has been viewed 279,399 times.
Tongue tricks can be a fun way to impress your friends. Some tricks are easy to learn, while others may require more control over your tongue muscles. With some practice, you can become skilled in performing cool tongue tricks.
Over time, the answer to the age-old question about the smallest thing in the universe has changed. In the past, people believed that grains of sand were the fundamental building blocks of everything we see around us.
Afterward, the atom was unearthed. The Greeks were the first to propose the idea of atoms, believing that objects could be endlessly divided into two until only one indivisible particle of matter remained. This incredibly tiny unit could not be further divided and was therefore named an “atom,” derived from the Greek word A-tomos. Where “A” means “not” and “tomos” means to divide.
It was once thought to be indivisible until it split open to uncover protons, neutrons, and electrons within. These too appeared to be fundamental particles until scientists discovered that protons and neutrons are composed of three quarks each.
So, what are the smallest particles in the universe?
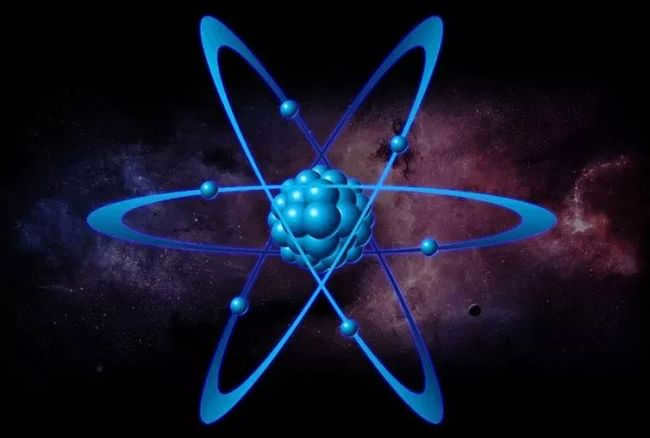

An electron is a subatomic particle with a negative charge. It can exist either as a free particle or as part of an atomic nucleus. Electrons within atoms are found in spherical shells of different sizes, which correspond to different energy levels. The larger the shell, the greater the energy of the electron. In electrical conductors, current is produced by the movement of electrons from atom to atom and from negative to positive electric poles. In semiconductor materials, current is also generated by the movement of electrons.
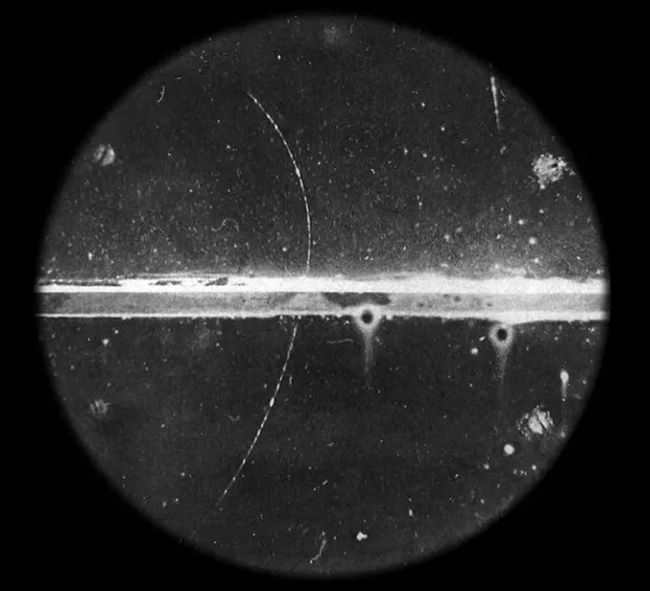
Positrons, which are the antiparticles of electrons, possess a positive charge as their main distinction. These positrons are created through the decay of nuclides that contain an excess of protons in their nucleus compared to the number of neutrons. When this decay occurs, these radionuclides emit a positron and a neutrino.
While the neutrino escapes without any interaction with the surrounding matter, the positron interacts with the electron. During this annihilation process, the masses of the positron and electron transform into two photons that move in nearly opposite directions.

The proton, a subatomic particle with a positive charge equal to that of an electron and a rest mass of 1.67262 × 10 -27 kg, is considered stable.
Around ten years ago, it appeared that both spectroscopy and scattering experiments were approaching a consensus on the proton’s radius, which was measured to be 0.8768 femtometers (millionths of a millionth of a millionth of a millimeter).
However, in 2010, a new discovery in spectroscopy disrupted this previously established agreement. The research team found that the proton’s radius was actually 0.84184 femtometers.
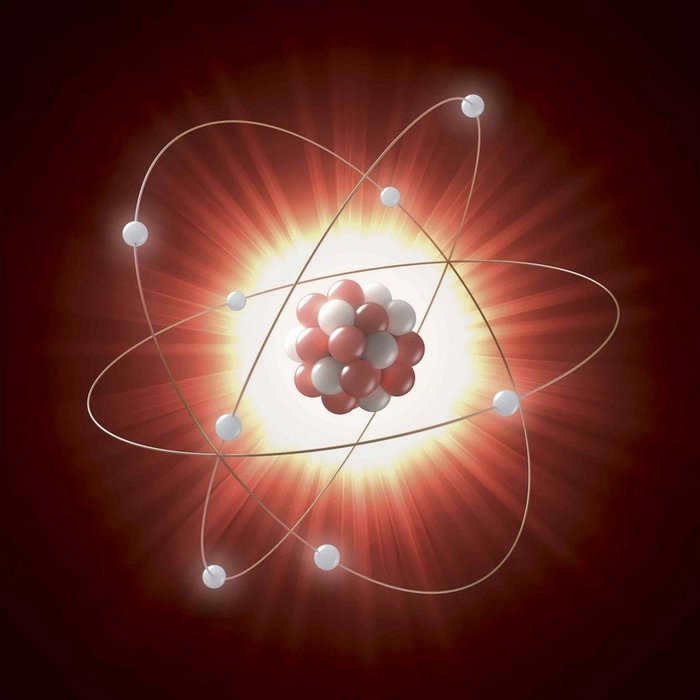
You may be aware that neutrons are present in the nucleus of an atom. In normal circumstances, protons and neutrons are closely bound together within the nucleus. However, during the process of radioactive decay, they can be dislodged from their position. The presence of neutrons can alter the mass of atoms, as they have a similar weight to that of a proton and an electron combined.
Neutrons can be found in almost all atoms, alongside protons and electrons. The only exception to this rule is hydrogen-1. Atoms with the same number of protons but varying numbers of neutrons are referred to as isotopes of the same element.
The chemical properties of an atom are not influenced by the number of neutrons it contains. Nevertheless, the number of neutrons does impact its stability, as measured by its half-life. A shorter half-life is characteristic of an unstable isotope, in which half of the atoms decay into lighter elements.
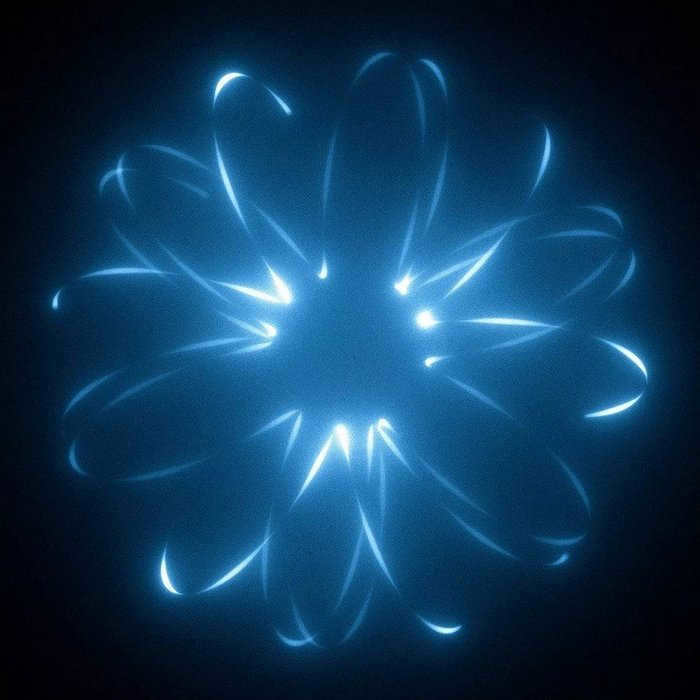
Envision a ray of golden sunlight streaming through a window. As indicated by quantum physics, this ray comprises of billions of minuscule bundles of light called photons that stream through the air. However, what precisely is a photon?
A photon is the most diminutive discrete sum or quantum of electromagnetic radiation. It is the fundamental unit of all light.
Photons are consistently moving and in a vacuum move at a steady speed to all observers of 2.998 × 10 8 m/s. This is typically alluded to as the speed of light, denoted by the letter c.
Per Einstein’s quantum theory of light, photons possess energy equivalent to the frequency of their oscillation multiplied by Planck’s constant. Einstein demonstrated that light is a flow of photons, where the energy of these photons corresponds to the magnitude of their oscillation frequency, and the brightness of light is determined by the quantity of photons present.

Quarks, which are fundamental particles in physics, join together to create hadrons like protons and neutrons, which are integral parts of atomic nuclei.
Quarks are held together, leading to the observation that quarks are never seen alone, but always in conjunction with other quarks. Consequently, it is not feasible to directly gauge characteristics such as mass, spin, and parity; these attributes must be deduced from the particles they form.
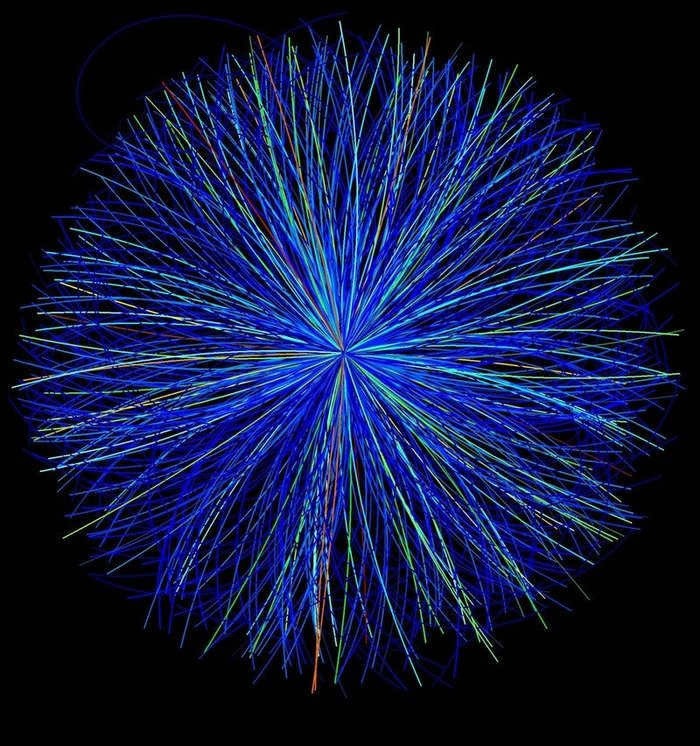
A mere fraction of a second after the occurrence of the Big Bang, the cosmos consisted of an extraordinarily compact plasma, so scorching that no atomic nuclei or even subatomic particles could subsist.
This plasma was composed of quarks, the fundamental constituents of nucleons and various other elementary particles, as well as gluons, massless particles that facilitate the exchange of force between quarks.
Gluons function as intermediary particles for the color force connecting quarks, similar to how photons enable the exchange of electromagnetic force between two charged particles. Thus, the gluon can be perceived as the fundamental exchange particle that underlies the strong interaction between protons and neutrons within the atomic nucleus.

Muons possess a negative charge similar to that of electrons, but they have a mass that is 200 times greater. These particles are created when cosmic rays, which are high-energy particles, collide with atoms in the Earth’s atmosphere.
Moving at a speed close to that of light, muons bombard the Earth from all directions. Approximately one muon strikes each region the size of a hand per second, and these particles can pass through hundreds of meters of solid material before being absorbed.
Christine Carloganou, a physicist at the Clermont-Ferrand Physics Laboratory in France, explains that muons are well-suited for imaging large and dense objects without causing any damage due to their widespread presence and ability to penetrate.
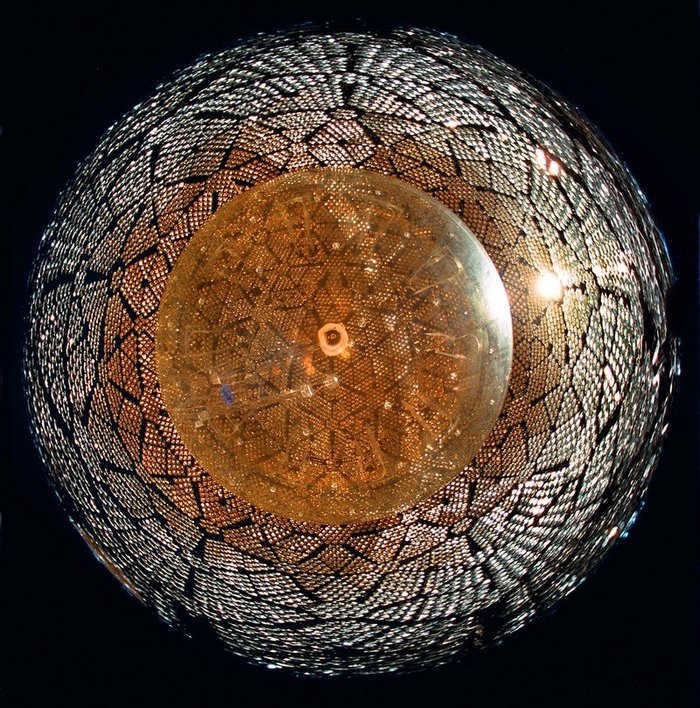
A neutrino is a subatomic particle that bears a strong resemblance to an electron, but lacks an electric charge and possesses an extremely minute mass, potentially even zero.
Neutrinos are among the most abundant particles in the cosmos. Nevertheless, due to their minimal interaction with matter, detecting them is an exceedingly arduous task.
The detection of neutrinos necessitates the use of exceedingly large and highly sensitive detectors. Typically, low-energy neutrinos traverse countless light-years of ordinary matter prior to engaging in any form of interaction.
Consequently, all terrestrial neutrino experiments are predicated on the measurement of a minuscule fraction of neutrinos that interact within reasonably sized detectors.
1- The Higgs Boson (“God Particle”)
Particle physics often struggles to attract the same level of attention as politics and celebrity gossip, but the Higgs boson has managed to generate significant interest. Perhaps it was the controversial and iconic nickname given to the boson, the “God Particle,” that kept the media abuzz.
Alternatively, the fascinating theory that the Higgs boson could be responsible for the entirety of the universe’s mass captures the imagination.
What is the smallest particle in terms of mass: A) proton B) electron C) neutron D) The masses of protons, neutrons, and electrons are equal.
An email has been sent to [email protected] containing a link to change your password.
If you do not see the email, please check your spam folder.
Please sign in or create an account to ask a question.
You must create an account on Uchit.ru
The platform formerly known as “Your Lesson” is now called Uchi.Answers. To access it, please use your Uchi.ru login credentials. If you don’t have an account yet, you can register on the platform.
A simplified explanation of the atom’s structure. Understanding the complex.
Atoms are the building blocks of everything in the world. But where do they come from, and what are they made of themselves? Today, we will answer these fundamental questions. Many people on Earth admit that they struggle to understand the structure of atoms, even though they are made up of them.
Of course, it is important to note that in this article, we will explain everything in the simplest and most engaging way possible, without overwhelming you with scientific terms. If you are interested in delving into this topic on a more advanced level, we recommend consulting specialized literature. Nonetheless, the information provided here can serve as a valuable resource for your studies and enhance your knowledge.
An atom is a tiny particle with minimal size and mass, constituting the basic building block of a chemical element and carrying its distinct properties. Put simply, it is the tiniest component of a substance capable of engaging in chemical reactions.
Discovery and Structure Overview
The notion of the atom has been present since ancient times in Greece. Atomism, a physical theory, posits that all material objects consist of indivisible particles. This concept also emerged concurrently in ancient India.
It is unclear whether the philosophers of that era were informed about the existence of atoms by extraterrestrial beings, or if they conceived of it on their own. However, it was not until the seventeenth century, when Europe emerged from the depths of the Inquisition and the Middle Ages, that chemists were able to experimentally validate this theory.
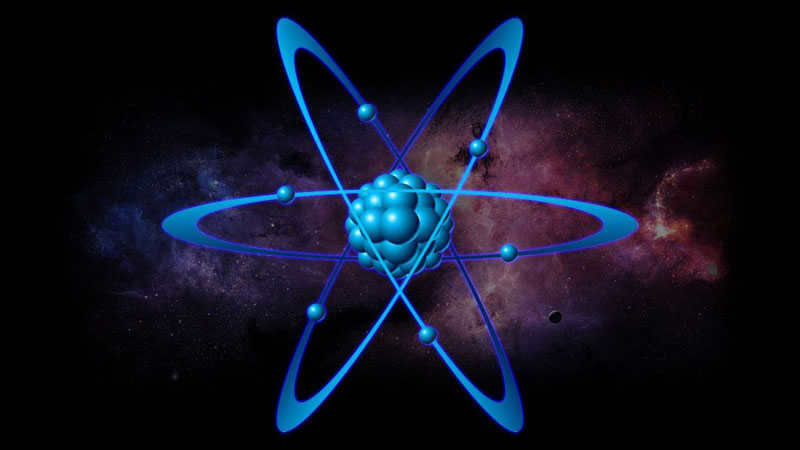
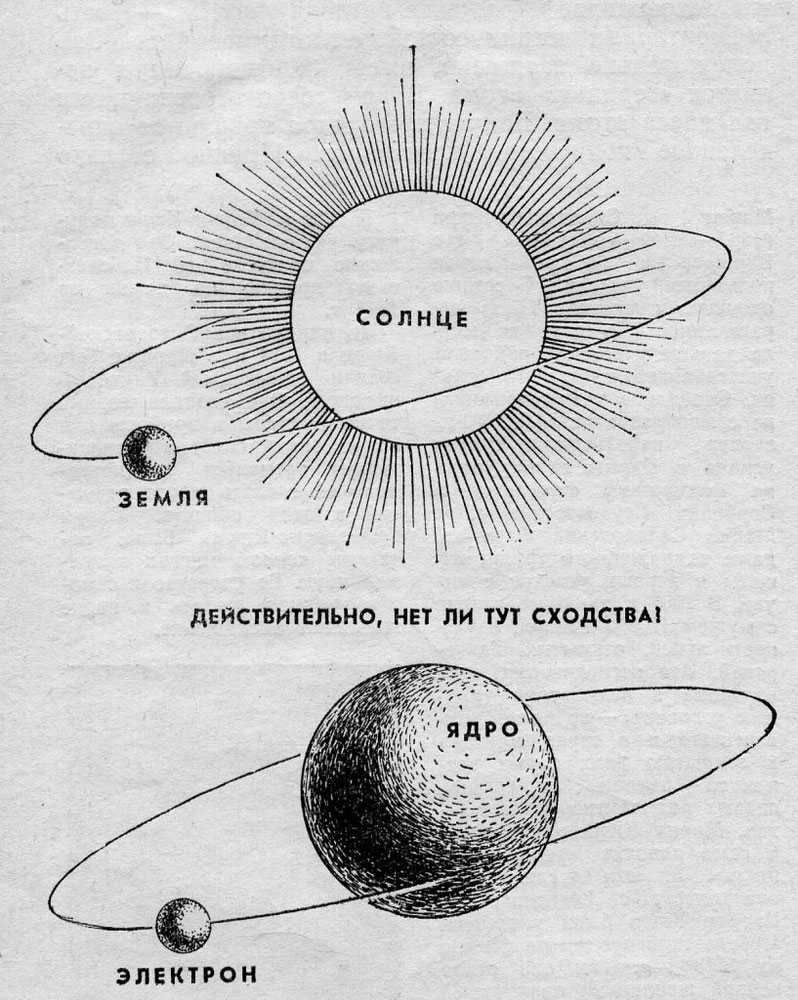
For a considerable period of time, the prevailing belief regarding the structure of the atom was that it was an indivisible particle. It was not until the early twentieth century that it became evident that the atom could be divided. Through his renowned alpha particle deflection experiment, Rutherford discovered that the atom is composed of a nucleus, around which electrons orbit. The planetary model of the atom was subsequently embraced, positing that electrons orbit the nucleus in a manner akin to the planets of our solar system orbiting a star.
There have been significant advancements in our understanding of the atomic structure. The atom is composed of subatomic particles called nucleons, which include protons and neutrons. These nucleons make up the majority of the atom’s mass. However, it is important to note that protons and neutrons are not indivisible particles themselves, but rather consist of fundamental particles known as quarks.
The atomic nucleus carries a positive electric charge, while the negatively charged electrons orbit around it. As a result, the atom remains electrically neutral.
Displayed below is a basic diagram illustrating the structure of a carbon atom.

Properties of atoms
Mass
Atoms are typically quantified in terms of their mass using atomic mass units (a.u.m.). The atomic mass unit is a measurement that represents the mass of 1/12th of a carbon atom in its ground state.
In the field of chemistry, the mass of atoms is often measured using the concept of a mole. A mole is defined as the amount of a substance that contains a number of atoms equal to Avogadro’s number.
Size
The dimensions of atoms are exceedingly minuscule. To illustrate, the tiniest atom is the Helium atom, measuring a mere 32 picometers in radius. On the other end of the spectrum, we have the cesium atom, which boasts a radius of 225 picometers. It is worth noting that the prefix “pico” denotes a factor of ten to the power of negative twelve! In other words, if we were to reduce a distance of 32 meters by a trillionfold, we would arrive at the radius of a helium atom.
Considering the immense scale of things, it is quite remarkable that the atom is comprised of 99% empty space. The nucleus and electrons, in comparison, occupy an infinitesimally small portion of its overall volume. To help visualize this concept, let’s use an analogy. If we were to envision the atom as an Olympic stadium in Beijing (or any other large stadium for that matter), the nucleus would resemble a cherry delicately perched at the very center of the playing field. Meanwhile, the electron orbits would hover around the upper levels of the stands. To add to the astonishment, imagine that this tiny cherry possesses a staggering weight of 30 million tons. Truly awe-inspiring, wouldn’t you agree?


If you envision the atom as a stadium, the nucleus would be comparable in size to a cherry positioned at the center of the field.
As you are aware, the Mendeleev table now serves as a classification system for the diverse range of atoms. Currently, there are 118 elements listed (and potentially 126 if including the predicted but undiscovered elements), excluding isotopes. However, this arrangement was not always the norm.

Therefore, one could argue that the constituent particles that compose our bodies were once components of ancient celestial bodies.
What prevents the decay of an atom’s nucleus?
In the realm of physics, there exist four fundamental interactions between particles and the entities they constitute. These interactions encompass the strong, weak, electromagnetic, and gravitational forces.
It is the strong force, which exhibits itself at the level of atomic nuclei and is accountable for the attraction between nucleons, that renders the atom remarkably resilient.
Relatively recently, it was discovered that the fission of atomic nuclei releases an immense amount of energy. The fission of heavy atomic nuclei serves as the primary source of energy in nuclear reactors and nuclear weapons.

Therefore, my dear companions, after acquainting you with the structure and fundamentals of the atom, we simply need to reiterate that our team of writers is always available to assist you. It doesn’t matter if you require assistance with a nuclear physics thesis or a small quiz – circumstances may vary, but there is always a solution. Consider the vastness of the universe, entrust your assignment to Zaochnik, and remember – there is no need to worry.

Currently, 58,742 educational institutions have access to exclusive cumulative discounts ranging from 2% to 25%. To determine the applicable discount for all staff members of your educational institution, please log in to your personal Infoworks account.


Professional training program
Teaching Physics: theory and methodology in educational institutions.
We can apply your educational institution’s discount to this offer (the amount will depend on the number of your colleagues who have already completed Infoworks courses).
Currently, there are additional cumulative discounts (ranging from 2% to 25%) available to 58,742 educational institutions. To determine the discount available for all staff members at your educational institution, please log in to your personal Infoworks account.


Enroll in our Professional Development Course
Physics Application: Techniques for Problem Solving
We are pleased to offer a discount for your educational institution, which can be combined with our current discount. The amount of the discount will depend on the number of your colleagues who have already taken courses with Infoworks.
Currently, 58,742 educational institutions are receiving additional discounts ranging from 2% to 25%. To find out the discount applicable to all employees of your institution, please log in to your personal Infoworks account.




Tips for Parents: Homeschooling Made Easy
Breakdown of the Presentation:


Assignment #1: Slide 3:
Who among the scientists listed below is credited as the originator of the special theory of relativity?

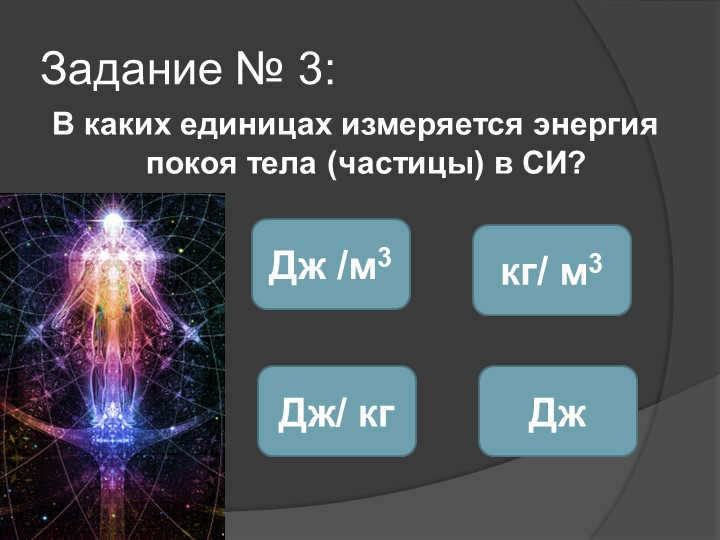
For the third assignment, let’s discuss the measurement of the resting energy of a body (particle) in the International System of Units (SI).

For the sixth slide of Assignment #4, the question is: Which particle does not possess any rest mass?

Slide number 7 for Assignment #5:
There is a body (a spacecraft) that is currently in motion at a speed of 0.95 s. At the exact same moment, the body’s longitudinal dimensions are…

Slide 8 Task #6:
The velocity of a spacecraft is 0.87 s. When the mass of the spacecraft, the mass of the astronauts, and the mass of the food supply all increase by a factor of 2, how will this affect the time it takes for the astronauts to utilize the food supply?
The time to utilize the food supply for the astronauts will:
- Not change
- Increase by a factor of 2
- Increase by a factor of √2
- Decrease by a factor of 2

Slide number 9:
When objects are heated, their mass remains constant and does not change.

Task #8 on slide 10:
If an elementary particle is moving at the speed of light, then…
the mass of the particle becomes zero
the particle possesses an electric charge
the particle cannot break down into constituent parts
the Earth’s gravitational field affects the particle.


Slide number 11 of Assignment #9:
What is the time required for light to travel from Earth to Mercury? The distance between Earth and Mercury is 58 million kilometers.
200 seconds
0.02 seconds
1000 seconds
100 seconds

Assignment #10:
What is the mass of a body that has a rest energy of 9*1013 J?
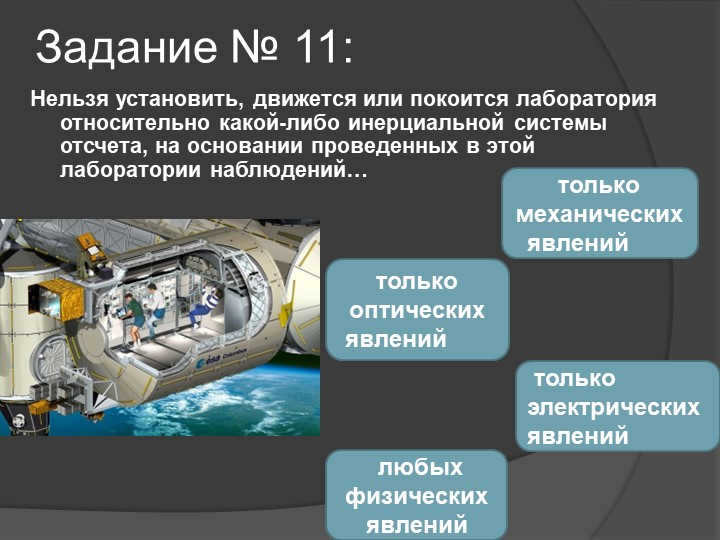

Slide 13 Assignment #11:
Based on the observations made in the laboratory, it is impossible to determine whether the laboratory is in motion or at rest relative to any inertial reference frame…
any physical phenomena
only mechanical phenomena
only optical phenomena
only electrical phenomena


Slide 14 Assignment #12:
The Sun consistently emits a substantial quantity of energy. The alteration in its mass and the emitted energy E are connected by the correlation:

Assignment #13:
In accordance with STO, the mass of a particle can be determined by the total energy and momentum of a body using the equation
m =√ E2/c4 – p2/c2. Which graph accurately depicts the relationship between the mass of a body in STO and the momentum of the particle?

Assignment #14: 16-slide – How many times will the density of the body change as it moves at a speed of 0.8 s?

Task number 15 on slide 17:
A calculation needs to be performed for:
A) determining the energy of a subatomic particle moving at a constant velocity
B) calculating the output of a nuclear reactor
C) assessing the power of a jet engine
The application of the principles or equations of Special Theory of Relativity (STO) is necessary only:
in the case of A
in the case of B
in the cases of A and B
in the case of C

Assignment #16: In a spaceship journeying towards a faraway star with an unchanging speed, a scientific investigation is being carried out to examine the interaction between charged spheres. If the study conditions are the same in both scenarios, will the outcomes differ from a similar study conducted on Earth?
No, the outcomes will remain identical regardless of the spaceship’s velocity.
Yes, because the spaceship is moving at a specific speed.
No, only at low spaceship velocities.
Yes, due to relativistic effects if the spaceship’s speed approaches the speed of light.

Slide 19 Task #17:
Within the installation, the occurrence of a spark discharge generates a burst of both light and sound, which is then detected by a sensor placed 1 m away from the arrester. The diagram illustrates the relative positioning of the arrester P and the sensor D. The time taken for light to travel from the arrester to the sensor is denoted as T, while the time for sound propagation is represented as t.
By conducting experiments using two units, namely 1 and 2, situated within a spacecraft in motion relative to the Earth, the astronauts observed the following:
T1 = T2, t1 = t2
T1 = T2, t1 > t2
T1 < T2, t1 < t2

Assignment #18, 20 slides:
When a ray of blue light hits a mirror that is moving in a vacuum with a velocity , pointing downward, relative to an inertial reference frame (ISO), the speed of light in this ISO after reflection from the mirror can be determined. The angle of incidence is 60°.
с
The speed of light in the ISO after reflection from the mirror is either C – 2v or C + 2v.

Slide number 21 Task #19:
A vehicle is stationary on the road with its front headlights illuminated. In this scenario, the magnitude of the velocity of light propagation with respect to the road is denoted as V. As per the postulate formulated by A. Einstein, when the vehicle moves along the road at a constant velocity, the magnitude of the speed of light propagation relative to the road:
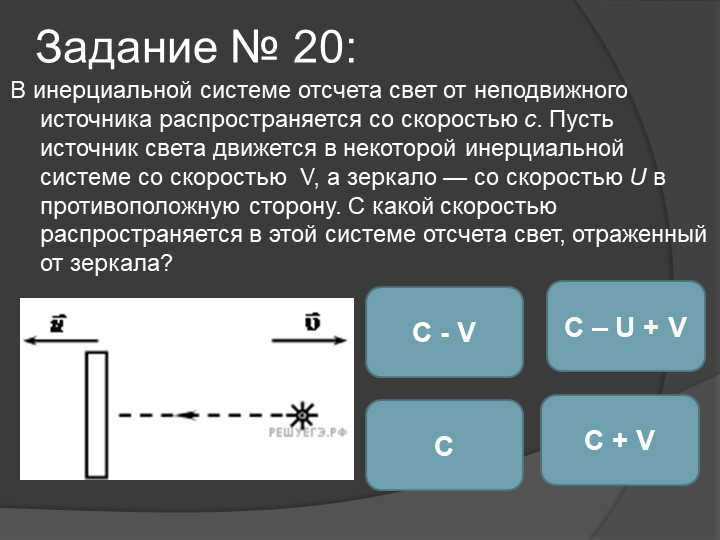
Slide number 22, Assignment #20:
In a frame of reference that is not accelerating, the speed of light coming from a source that is not moving is c. Suppose the source of light is moving in a system that is not accelerating with a velocity of V, and the mirror is moving in the opposite direction with a velocity of U. What is the speed at which the light is reflected from the mirror in this frame of reference?
C
C – V
C – U + V
C + V
Regrettably, while revolutionizing science with the concepts of space, time, energy, momentum, and mass, Einstein inadvertently created two contrasting definitions of mass, among other things. As a result, every statement we make can be interpreted in two distinct ways. However, within the realm of physics, there is no ambiguity. Experts understand precisely what we are referring to and can make accurate predictions and utilize appropriate equations. The issue lies in the interpretation of the word itself. Nonetheless, words hold significance, particularly when discussing physics with non-experts and students who have yet to grasp the full understanding of equations.
In my writings, when I refer to “mass,” I am specifically referring to a property of an object that is sometimes referred to as “invariant mass” or “rest mass.” For my colleagues in particle physics and myself, we simply refer to it as “mass.” The terms “invariant mass” or “rest mass” are only used to clarify the meaning of “mass” if there is a need to introduce a second quantity that is also referred to as “mass,” which is typically known as “relativistic mass.” Particle physicists avoid this confusion by not utilizing the concept of “relativistic mass” at all.
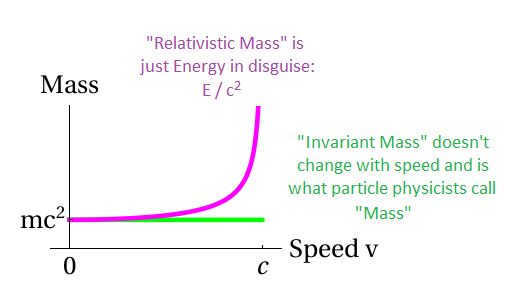
Observer dependence applies to both energy and momentum. It also applies to relativistic mass. This is because relativistic mass is calculated by dividing energy by a constant, which is denoted as c 2. Therefore, if we define mass as “relativistic”, different observers will have varying opinions on the mass of an object m, even though they all agree that E = mc 2.
However, there is a concept called rest mass, which I refer to as simply “mass”. This type of mass is independent of the observer and is sometimes referred to as invariant mass. All observers will agree on the mass of an object m when defined in this way. Additionally, all observers agree that if you are at rest relative to an object, the energy you measure will be mc 2. Conversely, if you are not at rest relative to the object, the energy measurement will differ significantly. In conclusion, the definition of mass used in my articles encompasses these principles.
– If the observer measures that the object’s velocity relative to them is v=0, the observer will determine that the object’s energy is equal to mc2 and its momentum is 0.
– However, if the object is moving relative to the observer, the observer will measure that the object’s energy is greater than mc2 and its momentum is also greater than 0 (p > 0).
– In the general case, the relationships between energy (E), momentum (p), mass (m), and velocity (v) can be described by two equations:
o v = pc/E
o
– These equations align with the previous statements: if p=0, then v=0 and therefore E = mc2, and if p>0, then v>0 and since pc > 0, E must be greater than mc2.
These equations and their graphical representation are discussed in more detail in another article.
I would like to provide you with the explanations as to why particle physicists employ these formulas and do not accept the notion that the equation E = mc 2 is universally valid. This formula specifically applies to situations where the observer remains stationary in relation to the object. To illustrate this, I will pose several inquiries, the responses to which differ significantly depending on the interpretation of the term “mass”. This will serve to highlight the significant challenges that arise when there are two conflicting definitions of mass and elucidate why it is more convenient to work with observer-independent mass in the field of particle physics.
Does a photon, a particle of light, possess mass or not?
According to my definition of mass, the answer is no. The photon is considered a massless particle, meaning its velocity is constantly equal to the universal speed limit, c. In contrast, an electron does possess mass, resulting in its velocity always being less than c. The mass of all electrons is measured at 0.000511 GeV/c².
Is the mass of an electron really greater than the mass of an atomic nucleus?
According to my definition of mass, the mass of an electron is never greater than the mass of the proton or neutron that makes up the nucleus. All observers would agree that the electron’s mass is 1800 times less than that of the nucleus.
However, if we consider relativistic mass, the answer is dependent on the situation. The mass of a stationary electron is less, whereas a high-speed electron would have a greater mass. It is even possible to arrange circumstances in such a way that the mass of the electron matches that of the chosen nucleus. In general, we can only conclude that the rest mass of the electron is less than the rest mass of the nucleus.
Are neutrinos endowed with mass?
From the 1930s, when neutrinos were initially postulated, until the 1990s, the presence of mass in neutrinos remained unknown, according to my understanding of mass. Presently, it is (almost certainly) established that neutrinos possess mass.
However, if we are referring to relativistic mass, then undoubtedly, we have known this fact since the inception of the neutrino concept. All neutrinos possess energy, and therefore, akin to photons, they possess mass. The only question that remains is whether there exists an invariant mass.
Is the mass of all particles of the same type, such as photons, electrons, protons, and muons, identical?
According to my understanding of mass, the answer to this question is affirmative. All particles of the same type possess the same mass.
However, if we consider relativistic mass, the answer is clearly negative. Two electrons with different velocities exhibit distinct masses. Their invariant mass remains constant though.
Is Newton’s old formula F = ma, relating mass, impact, and acceleration, true?
According to my understanding of mass, the answer is negative. In Einstein’s theory of relativity, this formula has been revised.
However, if by mass we are referring to relativistic mass, the truthfulness of the formula varies depending on the circumstances. If the force and motion vectors of the particle are perpendicular, then the formula holds true; otherwise, it does not.
According to my concept of mass, the response would be: negative. Take a look at the graph provided. While various observers might assign varying energies to the particle, they will all concur on its mass.
However, if by mass I am referring to relativistic mass, the answer is: affirmative. Different observers can assign distinct energies to the particle and, as a result, different masses. They will only reach an agreement on the invariant mass.
Therefore, it is evident that there exists a linguistic dilemma. If we fail to specify the precise definition of mass we are employing, we will obtain entirely divergent responses to the most basic inquiries in the field of physics. Regrettably, in the majority of texts aimed at the general public, and even in certain introductory university textbooks (!), the authors interchangeably employ these terms without providing any explanation. This has led to significant confusion among my readers, as they are presented with conflicting information about mass: one type of information pertains to rest mass, while the other pertains to relativistic mass. It is highly problematic to utilize a single term to refer to two distinct concepts.
It is, without a doubt, mere language. Language can be manipulated in any way you desire. Definitions and semantics hold no significance. When a physicist possesses equations, language is rendered an imperfect medium. Mathematics is never perplexing, and an individual who comprehends mathematics is never bewildered.
However, for the majority of individuals and novice students, it is a nightmare.
What can be done? One possibility is to insist on employing every conceivable term. Nevertheless, this approach would result in exceedingly confusing explanations.
– Energy of an object at rest = invariant mass multiplied by c 2 = relativistic mass multiplied by c 2
– Mass of an object in motion = invariant mass as previously mentioned, but energy = relativistic mass multiplied by c 2 it possesses more energy than before due to its motion.
This is excessively wordy. My colleagues and I are simply stating:
– An object at rest with a mass of m possesses energy E that is equivalent to mc2,
– and for an object in motion, the mass remains m, but the energy E is increased by the energy of its motion, surpassing mc2.
This approach maintains its significance while employing fewer distinct concepts and definitions, eliminating the need to contend with the conflicting interpretations of the term “mass”, one of which remains constant regardless of motion, while the other does not.
Linguistically, semantically, and conceptually, we should avoid using the term “relativistic mass” and eliminate the words “invariant” and “rest” from the definitions of “invariant mass” and “rest mass” because “relativistic mass” is a pointless concept. It is simply another term for the energy of a particle. Utilizing the concept of “relativistic mass” is akin to insisting on using the phrase “reddish-blue”. If I insist on using “reddish-blue” to describe raisins, you would object, as we already have a word for that color: purple. What is wrong with that? Additionally, you might argue, “Claiming that the color of raisins is a type of blue is incorrect and confusing. One might mistakenly believe that the color of a raisin is similar to that of the sky, but in reality, they are different.” Similarly, relativistic mass multiplied by c^2 is just another term for energy (which already has an appropriate word), and describing energy as if it were similar to mass only serves to confuse the reader.
The quantity on the right clearly does not require a new designation, as it is evidently neither E nor p – it is not conserved like E and p, but it is observer-independent (unlike E and p!)
The concept of “relativistic mass” did not emerge out of thin air or from some foolishness. It was introduced by Einstein himself, and not without reason, as he was concerned with the relationship between the energy of a system of objects and the mass of that system. However, while the idea of relativistic mass was popularized and spread by other renowned physicists of that era, Einstein himself seems to have abandoned this way of thinking, and not without cause. The contemporary community of particle physicists has done the same.
When writing articles and conducting research, I refrain from utilizing relativistic mass. Instead, I prefer to employ energy as it is a more accurate representation. For a particle itself, relativistic mass can be defined as simply the energy divided by c2. Additionally, when I refer to “mass,” I am always referring to the “invariant mass” or “rest mass,” which is agreed upon by all observers. The mass of an electron always remains constant at 0.000511 GeV/c2, regardless of its velocity. Furthermore, the mass of any electron is always smaller than the mass of an atomic nucleus. It is important to note that all photons in a vacuum are inherently massless. Lastly, the mass of the Higgs particle is constant at 125 GeV/c2, regardless of its speed. This linguistic and conceptual convention is commonly utilized by particle physicists. While it is not mandatory, it does help to avoid various practical and conceptual issues, as demonstrated here.
The weight of an African elephant, which is 6 tons, is 600 billion times greater than the weight of the smallest ant, which is 0.01 mg. The top quark is approximately the same number of times heavier than a neutrino. The reason for the significant difference in the masses of elementary particles remains unclear. Scientists are still searching for the Higgs boson, which will provide evidence of the existence of a field called the Higgs field that imparts mass to all particles in the Universe.

Most individuals possess knowledge regarding the concept of mass. It is universally understood that an ant is significantly smaller and lighter compared to an elephant. Even in the absence of gravity, the colossal pachyderm possesses greater mass: it is more difficult to manipulate and accelerate. Evidently, the elephant is more massive due to its composition of a significantly larger number of atoms in comparison to the ant. However, how are the masses of individual atoms determined? What can be inferred about the mass of the elementary particles that constitute them? Where does it originate from?
The issue of mass is characterized by two separate components. Firstly, there is a desire to comprehend the origins of mass itself. It is evident that there are at least three distinct mechanisms involved in its emergence, which will be outlined in the subsequent discussion. The fundamental role in the realm of physical theories pertaining to mass is attributed to the Higgs field, which is believed to permeate the entirety of the tangible world. It is postulated that elementary particles acquire mass through their interaction with this field. Should this conjecture hold true, the theory posits the existence of a corresponding particle – the Higgs boson, which scientists are tirelessly pursuing utilizing particle accelerators.
Furthermore, researchers are interested in understanding the reason behind the specific mass values associated with various elementary particles, where the heaviest particle is 11 orders of magnitude greater in mass compared to the lightest. To put it into perspective, an elephant is 11 orders of magnitude heavier than the tiniest ant.refer to Fig. The masses of the Standard Model particles.)
Therefore, what exactly is mass?
AN OVERVIEW OF THE PHYSICS OF THE HIGGS FIELDS
- Mass is a well-known characteristic of matter, but it still holds many enigmas for scientists. One of the primary questions that remains unanswered is how elementary particles obtain mass and why each particle has a distinct value.
- Discovering the answers to these inquiries will assist theorists in refining and expanding the Standard Model of particle physics, which serves as the foundation for understanding the fundamental laws of nature. The Extended Standard Model will also aid in unraveling the enigma of dark matter, which accounts for approximately 25% of the Universe.
- According to theoretical physics, elementary particles gain mass through their interaction with the pervasive Higgs quantum field that exists throughout the entire Universe. Experiments conducted at particle accelerators will serve to validate the existence of this field.
The concept of mass has evolved significantly from Newton’s definition to the current understanding based on the Standard Model. This modern understanding incorporates the use of a mathematical function known as the Lagrangian, which describes the interactions between different particles. By applying the principles of relativistic quantum theory, physicists can use the Lagrangian to calculate the behavior of elementary particles and how they combine to form protons and neutrons. Both elementary and composite particles are governed by the equation $F=ma$, which relates force, mass, and acceleration. The Lagrangian allows us to determine the value of m, representing the mass of the particle, and this value is not limited to Newton’s Second Law. According to the theory of relativity, particles without mass in a vacuum travel at the speed of light, while particles with mass move at slower speeds. By knowing the mass, we can calculate the speed of these particles. Additionally, the force of gravity acts on mass in the same way as it does on equivalent energy. The value of m, calculated using the Lagrangian, accurately represents the role of mass in all physical equations without exception.
Elementary particles possess a well-defined rest mass (particles lacking rest mass are referred to as massless). The aggregate mass of a composite particle is composed of the combined rest masses of its component particles, along with their kinetic energy of motion and potential energy of interaction. The relationship between energy and mass is elucidated by the renowned Einstein equation: $E=mc^2$, with c representing the speed of light. An exemplification of energy contributing to mass is observable in a familiar form of matter – protons and neutrons, which constitute atomic nuclei. These particles account for 4-5% of the mass and energy of the Universe (refer to box COSMIC INVENTORIZATION). As per the Standard Model, protons and neutrons are constituted by quarks that are bound together by massless gluons. Despite the fact that the components of each proton engage in a continuous waltz, we perceive it as a singular entity possessing an intrinsic mass equivalent to the total mass and energy of its constituent elementary particles.
According to the equations of the Standard Model, the majority of the mass of protons and neutrons can be attributed to the kinetic energy of quarks and gluons, with the remaining mass being the rest masses of the quarks. Therefore, approximately 4-5% of the entire universe, or the matter that we are familiar with, is comprised of the energy from the movement of quarks and gluons within protons and neutrons.
The Higgs mechanism
In contrast to protons and neutrons, elementary particles such as quarks and electrons are considered indivisible. The source of their rest masses remains a mystery in terms of mass origin. According to current physical theory, the masses of fundamental particles are a result of their interaction with the Higgs field. However, why does this field exist everywhere in the universe? Why does its strength not equal zero on a cosmic scale, as is the case with the electromagnetic field? What exactly is the Higgs field?
The Higgs field, being a quantum field, shares the same nature as other elementary particles such as electrons and photons. While the electromagnetic field is associated with the photon, the Higgs field possesses its own unique qualities that set it apart.
THE CHARACTERISTICS OF THE MYSTERIOUS HIGGS PARTICLE
THE PROCESS OF MASS GENERATION BY THE HIGGS FIELD.

“The Higgs field occupying what would otherwise be “empty” space can be likened to a sandy shore bustling with the joyful energy of children at play.”

The appearance of the particle as it passes through the area of the universe resembles that of a seller of frozen treats.

A group of youngsters encircle the frozen dessert trolley and bring it to a halt. Consequently, it gains “weight”.
INFUSING ACTUALITY
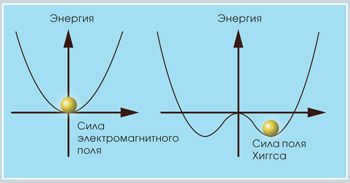
The energy of usual fields (for example, electromagnetic) is minimal at zero field strength (on the left). The cosmos resembles a ball that rolls up and halts at the bottom of a depression where the strength is zero. The Higgs field energy is minimal at field strengths different from zero (on the right). Consequently, in the state of lowest energy, the cosmos is saturated with the Higgs field.
THE PRODUCTION OF TWO PHENOMENA
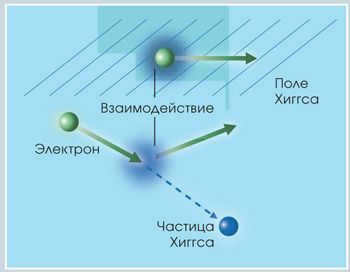
The same interaction is responsible for two distinct phenomena – the particle acquiring mass (top) and the emergence of the Higgs boson (bottom). This fact will be utilized in the experimental confirmation of the Higgs mechanism.
INTERACTION WITH OTHER PARTICLES
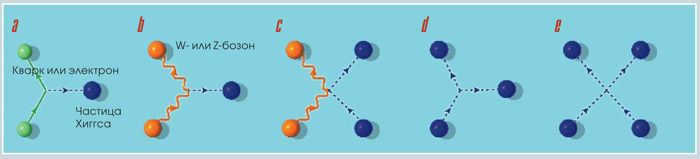
Feynman diagrams illustrate the interaction between the Higgs boson and other particles. Diagram a depicts the emission or absorption of the Higgs boson by a particle such as a quark or electron. Diagram b showcases the corresponding process involving the W- or Z-boson.
W- and Z-bosons have the ability to simultaneously interact with two Higgs bosons, as illustrated in diagram c. This diagram also represents the W- or Z-scattering of the Higgs boson, which can be thought of as a collision with it. The interactions shown in diagrams a, b, and c are what give rise to the masses of particles. Additionally, the Higgs bosons can interact with themselves, as shown in diagrams d and e. By combining multiple copies of the elementary diagrams, more complex processes can also be depicted. The interactions depicted in diagrams d and e are what determine the shape of the energy graph, which can be seen in the top left corner.
One of the categories can be described as “technical”. Each field is identified by its own spin, which is the angular momentum value of the corresponding particles. For instance, electrons have a spin of 1/2, while particles involved in interactions, such as photons, have a spin of 1. The Higgs boson has a spin of zero, which allows its field to be included in the Lagrangian in unconventional ways, resulting in its unique characteristics.
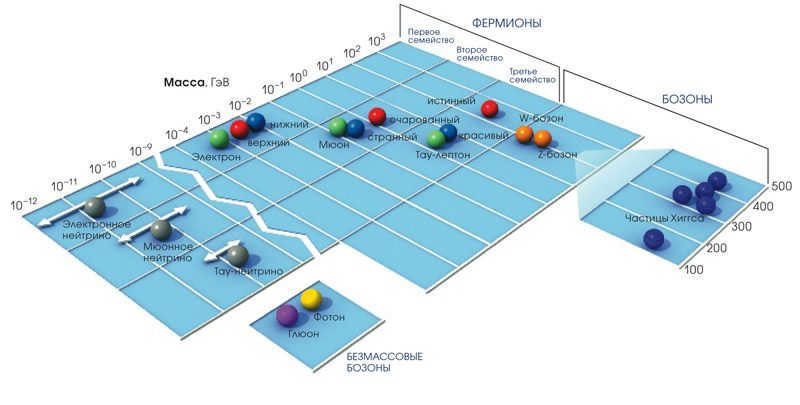
The masses of particles in the Standard Model vary greatly, differing by a factor of 11, and are a result of their interactions with the Higgs field. It is believed that there are at least five distinct types of Higgs bosons. Although the exact masses of these particles are unknown, the illustration provides a range of possible values.
The second distinctive characteristic of the Higgs field provides an explanation for why its strength varies from zero in all locations. Any system, including the entirety of the Universe, tends towards its lowest energy state, much like a ball rolling down into a depression. In the case of regular fields such as electromagnetic fields, the lowest energy state is represented by a strength of zero, indicating the absence of the field. If the field deviates from zero, the energy contained within it increases the overall energy of the system. However, for the Higgs field, the energy of the Universe is at its minimum when the strength is not zero. Consequently, for regular fields, the lowest point in the depression corresponds to a strength of zero; whereas for the Higgs field, there is a hill at the center of the depression (at zero strength), and the lowest points create a moat (cf. PROPERTIES OF THE ELUSIVE HIGGS PARTICLE). Similar to a ball, the Universe “rolls” into a circular depression, which signifies a non-zero field. Therefore, in its lowest energy state, the Universe is permeated by the Higgs field everywhere.
One unique aspect of the Higgs field is its interaction with other particles. It causes them to appear as if they possess a mass that is determined by the strength of the field and the force of interaction. The masses of the particles are determined by the terms in the Lagrangian that describe their interaction with the Higgs field.
However, the exact number of Higgs fields is still unknown. While the Standard Model states that all elementary particle masses are a result of a single Higgs field, it is time to replace it with a more comprehensive theory. The leading contenders for this replacement are the Supersymmetric Standard Models (SSM), which extend the Standard Model. In these models, each particle in the Standard Model has a superpartner (yet to be discovered) with similar properties (see “Dawn of a new era”, In the World of Science, No. 9, 2003.). The CCM requires at least two different types of Higgs fields, which interact with particles in the Standard Model to give them mass. These fields also contribute to the mass (though not entirely) of the superpartners. The two Higgs fields give rise to five types of Higgs bosons: three are electrically neutral, and two are charged. The masses of neutrinos are much smaller than the masses of other particles and may be a result of indirect interactions with these fields or be connected to a third type of Higgs field.
Furthermore, the Standard Model has undergone extensive testing and validation, confirming the accuracy of its various components. The intricate nature of this theory means that altering one element, such as the Higgs field, would have far-reaching consequences for the rest of the model. A prime example of this interconnectedness is the successful prediction of the top quark’s mass based on measurements of the W- and Z-bosons. This anticipation would have been flawed if the Higgs mechanism had deviated from its currently accepted form.
Furthermore, the Higgs mechanism is perfectly suited to elucidate the source of the masses of all particles in the Standard Model, including W- and Z-bosons, as well as quarks and leptons. Other alternative theories generally fall short in this regard. Additionally, the SSM provides a framework for constructing a unified comprehension of all the fundamental forces in nature. Lastly, the SSM helps to clarify why the energy basin of the Universe takes on the specific shape required for the Higgs mechanism. In the fundamental Standard Model, the shape of the basin must be assumed as a postulate, while in the SSM it is derived mathematically.
Experimental validation
It is the natural desire of physicists to confirm that mass is a consequence of interactions with the various Higgs fields. There are three key aspects that can be examined in order to do so. Firstly, it is necessary to search for Higgs bosons: if they are not found, the explanation cannot be deemed accurate. Currently, physicists are conducting a search for Higgs bosons at the Tevatron Collider at Fermi National Laboratory.
Secondly, once Higgs bosons are discovered, we will have the opportunity to observe how they interact with other particles. The characteristics of these interactions are determined by the terms of the Lagrangian, which also dictate the masses of the particles. Therefore, their existence can be experimentally verified, as the interaction forces and particle masses are directly linked.
COSMIC INVENTORY
The theory of the Higgs field explains how elementary particles, which are the smallest “building blocks” of the Universe, gain mass. However, the Higgs mechanism is not the sole source of mass-energy in the Universe. The concept of “mass-energy” combines mass and energy, which are connected by Einstein’s formula $E = mc^2$.
About 70% of the mass-energy in the Universe is concentrated in dark energy, which is not directly associated with particles. The primary indication of the existence of dark energy is the acceleration of the Universe’s expansion. The nature of dark energy remains one of the most challenging questions in modern physics (refer to “Cosmic Enigma”, “In the World of Science”, No. 12, 2004).
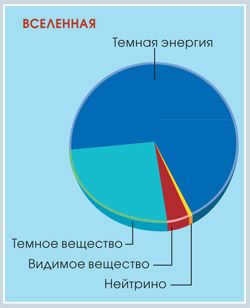
Approximately 30% of the total mass-energy in the Universe is composed of particles known as matter. These particles, such as protons, neutrons, and electrons, are responsible for about one-sixth of all matter, which equates to approximately 4-5% of the entire universe. The majority of this mass is attributed to the kinetic energy of quarks and gluons that move within protons and neutrons. Additionally, a smaller portion of the Universe’s matter is comprised of neutrinos, which can be classified into three groups and possess minuscule masses. Although the exact masses of neutrinos have yet to be determined, current data suggests that their maximum value is no more than 0.5% of the total mass of the Universe.
Dark matter, which makes up 25% of the mass-energy of the Universe, remains invisible to us but its presence is evident through its gravitational effects on the objects we observe. The exact nature of dark matter is still unknown, but there are promising candidates that are currently being tested through various experiments (refer to “The Search for Dark Matter,” Science World, No. 7, 2003). It is believed that dark matter is composed of massive particles, as its gravitational influence allows it to form clumps on a galactic scale. The multitude of evidence suggests that dark matter cannot be made up of ordinary particles from the Standard Model.

The primary contender for the title of dark matter particle is the lightest superpartner (LSP). It is present in extensions of the Standard Model known as Supersymmetric Standard Models (SSM). Evidently, the mass of the LSP is approximately equivalent to 100 masses of protons.
Moreover, the Standard Model and the SSM involve distinct sets of Higgs fields that correspond to distinct sets of bosons with unique characteristics. Scientists require colliders that can generate sufficient collision energy to produce various Higgs bosons and sufficient intensity to create them in large quantities. Additionally, these colliders must be equipped with highly effective detectors to analyze the resulting particles.
The search for the Higgs boson is made difficult by the fact that we must investigate a broad spectrum of masses, as the exact masses of the Higgs bosons have not yet been determined.
Due to theoretical calculations and analysis of experimental data, we can only make a rough estimation of the expected mass.
Scientists were unable to find any evidence of the Higgs boson, a particle that should weigh at least 120 times more than a proton, during experiments at the Large Electron-Positron Collider (LEP) at CERN. Despite many precise measurements and data collected at other colliders, such as the Tevatron and the Stanford Linear Accelerator Center, no direct confirmation of the Higgs boson’s existence was found before LEP closed in 2000. However, the data collected did support the theory that the Higgs boson exists, as long as it is no heavier than 200 proton masses and interacts with other particles in specific ways. This upper limit on the boson’s mass helps narrow down the search for its existence.
Dark matter
Studying Higgs bosons is not only important for understanding the origin of mass, but it also holds the key to unraveling the mystery surrounding dark matter. In the context of dark matter theories, the lightest superpartner (LSP) plays a crucial role. Most superpartners undergo rapid decay, eventually leading to the creation of LSP. The stability of LSP arises from the fact that there are no lighter particles for it to decay into. (A superpartner cannot decay solely into Standard Model particles; at least one of the decay products must be a superpartner.) These superpartner particles likely emerged in the early stages of the Big Bang but quickly transformed into LSP, thus forming the basis of dark matter.
The presence of Higgs bosons can also have a direct impact on the quantity of dark matter present in the Universe. It is understood that the number of LSPs (lightest supersymmetric particles) in existence today must be smaller than immediately following the Big Bang, as some LSPs may have collided and annihilated into quarks, leptons, and photons. The rate of annihilation could potentially be higher for LSPs that interact with Higgs bosons.
As previously mentioned, the two primary SSM (Standard Model) Higgs fields are responsible for granting mass to particles within the Standard Model, as well as contributing some mass to superpartners like LSPs. The remainder of the mass is obtained through additional interactions with other Higgs fields or their equivalents. Theoretical models outlining these processes have been developed in general terms, although the specific details will only be known once we gather data about the superpartners themselves. It is anticipated that such information will be obtained through experiments at the LHC (Large Hadron Collider) or potentially even the Tevatron.
The masses of neutrinos could also arise from interactions with additional Higgs fields. Previously, it was believed that neutrinos had no mass, but in 1979, theorists made a prediction that they do possess extremely small masses. Over the past decade, multiple significant experiments have confirmed these predictions (see “Unraveling the Mystery of Solar Neutrinos,” Science World, No. 9, 2003.). Neutrinos are a million times lighter than electrons, the second lightest particles. Due to their lack of electric charge, it is more challenging to theoretically explain the origins of their masses compared to charged particles. The mass of each type of neutrino is influenced by several processes, and for technical reasons, its precise value is determined through the solution of an equation rather than a simple addition of terms.
Therefore, we have identified three different ways in which mass manifests itself: the fundamental and well-known type of mass (which pertains to protons, neutrons, and consequently atoms) arises from the movement of the quarks that compose protons and neutrons. The mass of a proton would remain relatively unchanged even in the absence of the Higgs field. However, the masses of quarks and electrons are entirely contingent upon the presence of the Higgs field; without it, their masses would effectively be reduced to zero. Lastly, the majority of the mass exhibited by superpartners, and therefore the mass of dark matter particles, if indeed composed of the lightest superpartners, can be attributed to additional interactions.
Finally, let’s delve into the issue of particle families. Over the past 50 years, physicists have unveiled that our observable universe is comprised of merely six particles: three matter particles (top quarks, bottom quarks, and electrons), two quanta that generate the forces of interactions (photons and gluons), and Higgs bosons – an extraordinary and astonishingly simple depiction. However, there are an additional four quarks, two electron-like particles, and three types of neutrinos that have been identified. These particles have very brief lifespans or feeble interactions with the other six particles. As a result, three distinct families emerge: 1) top (u) and bottom (d) quarks, electron neutrino, and electron; 2) charmed (c) and strange (s) quarks, muon neutrino, and muon; 3) true (t) and beautiful (b) quarks, tau neutrino, and tau lepton. The interactions among the particles within each family are identical, with the only difference being that they are stronger in the second family compared to the first, and even stronger in the third family. Since the masses of the particles are determined by the Higgs field, these particles must interact with it in distinct ways.
Therefore, the issue regarding families presents two inquiries. Why do we have three families when one seems sufficient to explain the observable world? And why do particles from different families have varying masses, with each mass being exactly what it is? It is not surprising that physicists are endeavoring to comprehend why nature consists of three nearly identical particle families. They aim to gain a complete understanding of the laws of nature, its fundamental particles, and forces. We require a theory that encompasses all particles and their mass ratios, without any prior assumptions about the magnitude of the masses or the use of fitting parameters. If the presence of three families holds significance, it is the key that has yet to be fully realized.
The Standard Model and the SSM can acknowledge the observed configuration of the families, but are unable to provide an explanation for it. It is not claimed that the SSM has not yet elucidated the family structure, but rather that it is incapable of doing so entirely. The significance of string theory lies not in its ability to propose a quantum theory encompassing all forces, but rather in its capacity to clarify the nature of elementary particles, the existence of three families, and the varying interactions of these families with the Higgs field. It allows for the emergence of distinct families that are not identical. These differences are described by properties that do not affect the strong, weak, electromagnetic, and gravitational forces, but rather impact the interaction with the Higgs fields and correspond to three families with different masses. String theory allows for numerous possible family structures, and the reason why nature has chosen the one we observe and not another remains unknown (cf. “The Landscape of String Theory,” In the World of Science, no. 12, 2004). Data on the masses of quarks, leptons, and their superpartners will aid in further investigating string theory.
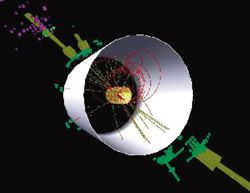
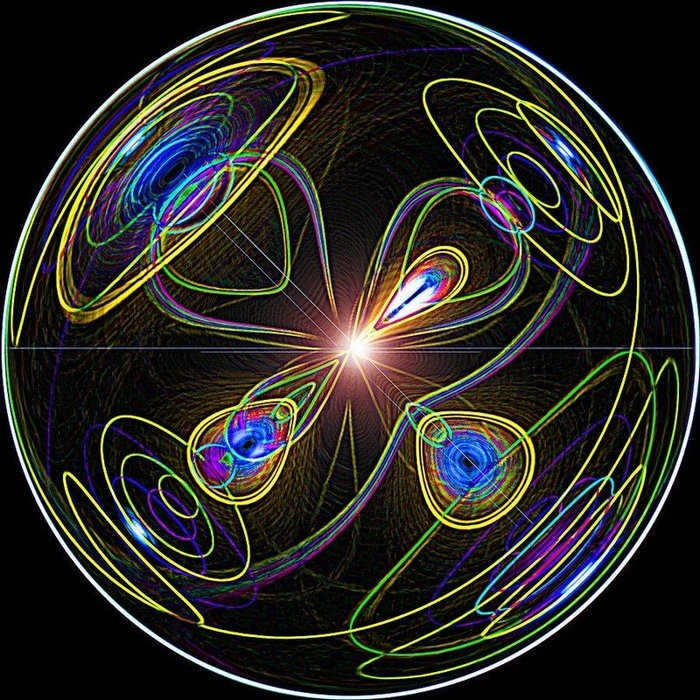
It is feasible that the interaction between a high-energy positron and an electron in the L3 detector of the Large Electron-Positron Collider (LEP) at CERN generated the Higgs boson. The traces of the particles are represented by the lines. The quantities of energy absorbed in the detector layers from particles moving away from the reaction are depicted by the green and purple blobs and gold histograms. Only by aggregating numerous events of this nature can physicists reach a conclusion as to whether Higgs bosons were present in any of the reactions or if all the events were caused by other reactions that merely imitated the signals emitted by Higgs bosons.
Scientists struggled for a long time to comprehend the essence of mass. It wasn’t until the emergence of the Standard Model of particle physics and the advancements in quantum field theory that physicists were able to formulate the right questions. Despite the fact that the origins and sizes of masses are still enigmatic, progress has been made in understanding the underlying structure. The concept of mass could only be grasped after the introduction of the Standard Model, the SSM, and string theory. Whether these theories will offer conclusive explanations remains to be seen. In any case, mass has become a prevalent subject of investigation in the field of particle physics.





